When you pile up cubes on each other, you can make all kinds of shapes and constructions. In the figures below cubes are piled up in the shape of a regular eight sphere. The more cubes you uses the more the construction looks like a real octahedron.

The principle of piling up cubes can be used in puzzles, especially puzzles in the shape of a cube are useful for piling up. An example of such a puzzle is the Ball in the Cage Puzzle. If you consider this puzzle as a cube you can use its type of construction for a lots of different puzzles. Below two examples are shown.

The first puzzle consist in fact of seven Imprisoned ball puzzles and is made with 48 rods. Twelve rods with a size of 24 x 2 x 2 units and 36 rods with a size of 12 x 2 x 2 units. The second Octacube puzzle is a size bigger...

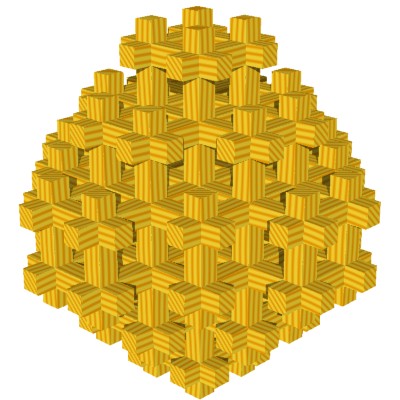
This puzzle is made of 72 pieces. twelve rods with a length of 32 x 2 x2 units, 24 pieces of 24 x 2 x 2 units and the remaining 36 rods have a length of 12 x 2 x2 units.

You can find numerous possibilities for making these constructions. When you understand the principle of the Imprisoned Ball puzzle, it isn't difficult to design an Octacube puzzle by yourself. The Tetracube puzzles are constructed with the same kind of principle as discribed here.