Design by Peter Query

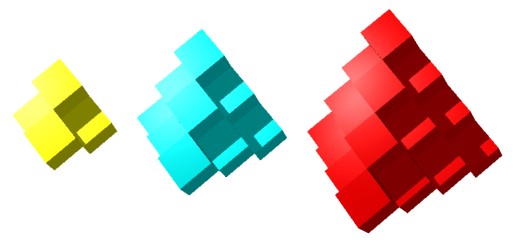
At the description of the Octacubes you could see how cubes can piled up to the shape of an octahedron. Cubes can also be put together in the shape of a pyramid as shown in the figures below. The problem with these constructions is that the cubes in the corners actually float in the air and fall of the structure if you try to make them.

It is possible to make the structures above by using the Ball-in-the-Cage Puzzle as base the same way as used in the Octacubes. The results of thes constructions are shown in the pictures below. The first puzzle consist of four cubes, the second of eleven and the last construction is made with 24 cubes.

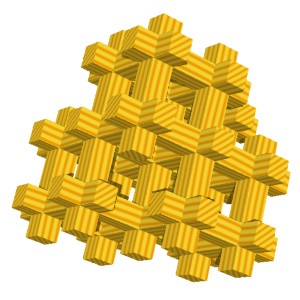
The smallest Tetracube puzzle can be made with 27 rods. Fifteen rods with a size of 18 x 2 x 2 units and twelve rods with a length of 18 x 2 x 2 units.

The next Tetracube is made out of 48 rods in three different lengths. Twelve rods are 24 x 2 x 2 units long, 24 rods with a size of 18 x 2 x2 units and twelve rods with a length of 12 x 2 x 2 units.

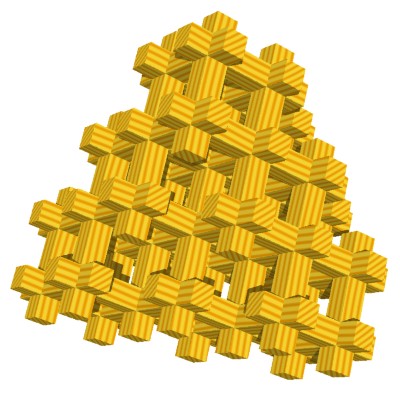
The last Tetracube shown here is constructed with 75 pieces. Fifteen pieces with a length of 30 x 2 x 2 units, Twelve rods with a size of 24 x 2 x 2 units, 36 rods with a length of 18 x 2 x 2 units and the remaining twelve rods have once again a size of 12 x 2 x 2 units.

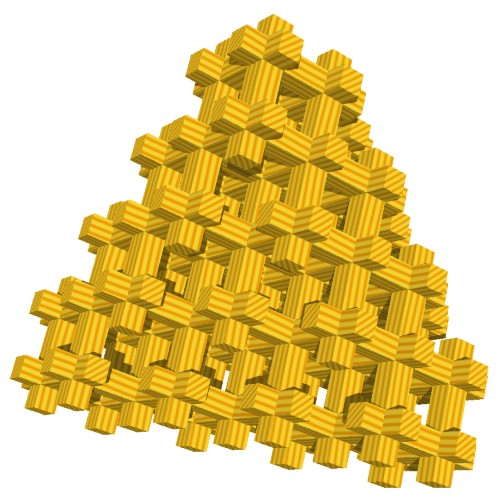
As with the Octacubes different possibilities exist in constructing the Tetracubes. The challenge is to design the most complex puzzles with the most less key pieces. All the cages can be filed with a ball or marble but you can also keep the cages empty.